第401题

参考答案：\(-\frac {7} {4}\)

第402题

参考答案：由题意可得：\(\begin{cases}
1 + a + 4 > 0 \\
{3^2} + 3a + 4 \leqslant 0 \\
\end{cases}\) \( \Rightarrow \{ a| - 5 < a \leqslant - \frac{{13}}{3}\} \)

第403题

参考答案：\(A = \{ x|{x^2} + ax + 4 > 0\} \) 设 \(g(x) = {x^2} + ax + 4\)\(\Delta = {a^2} - 16\)① 当 \(\Delta < 0,\) 即 \( - 4 < a < 4\) 时\(A = R,{\rm{ }}(0, + \infty ) \in A\) 成立② 当 \(\Delta = 0,\) 即 \(a = \pm 4\)时若 \(a = 4\)则 \(A = \{ x|x \ne - 2\} ,{\rm{ }}(0, + \infty ) \in A\) 成立若 \(a = - 4\) 则 \(A = \{ x|x \ne 2\} ,{\rm{ }}(0, + \infty ) \in A\) 不成立③ 当 \(\Delta > 0,\)即 \(a < - 4或a > 4\) 时即 \(A = \{ x|x > {x_1}{\rm{ }}\) 或 \(x < {x_2}\} \)若 \(a < 0,\)则 \({x_1} > 0,{x_2} > 0\) \(\therefore (0, + \infty ) \in A\) 不成立若 \(a > 0,\)则 \({x_1} < 0,{x_2} < 0\) \(\therefore (0, + \infty ) \in A\) 成立即 \(a > 4\)综上所述：\(a > - 4\)

第404题

A.\( -2\)

B.\( -1\)

C.\( 2\)

D.\( \frac{1}{2}\)

参考答案：C

第405题

A.\( \frac{1}{9}\)

B.\( \frac{\sqrt{3}}{2}\)

C.\( \sqrt{3}\)

D.\( 9\)

参考答案：A

第406题

A.\( a>b>c\)

B.\( b>a>c\)

C.\( a>c>b\)

D.\( c>a>b\)

参考答案：B

第407题

A.

B.

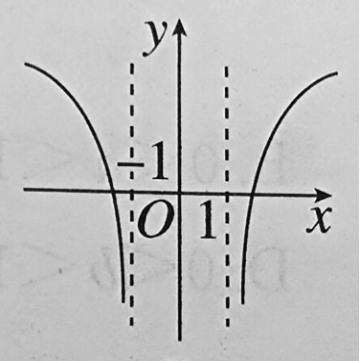
C.

D.

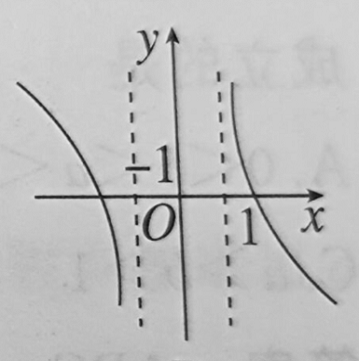
参考答案：B

第408题

A.

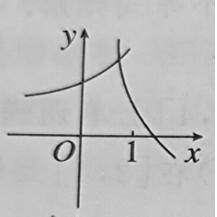
B.

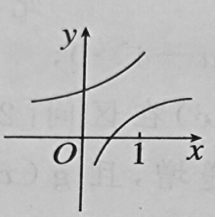
C.

D.

参考答案：D

第409题

A.\( (-\infty ,-3)\)

B.\( (-\infty ,-3)\cup (1,+\infty )\)

C.\( (-\infty ,-1)\)

D.\( (1,+\infty )\)

参考答案：D

第410题

A.\( (\frac{1}{3},+\infty )\)

B.\( (\frac{1}{3},\frac{2}{3}]\)

C.\( (1,+\infty )\)

D.\( (\frac{1}{3},\frac{2}{3})\cup (1,+\infty )\)

参考答案：D

第411题

A.\( (-\infty ,0)\)

B.\( (0,+\infty )\)

C.\( (-\infty ,{\mathrm{log}}-{a}3)\)

D.\( ({\mathrm{log}}-{a}3，+\infty )\)

参考答案：A

第412题

A.\( (-\mathrm{2,4}]\)

B.\( [-\mathrm{2,4}]\)

C.\( (-\infty ,4]\)

D.\( [4,+\infty ）\)

参考答案：A

第413题

A.\( f\left(x\right)={\mathrm{log}}-{2}x\)

B.\( f\left(x\right)=x+1\)

C.\(f\left ( {x} \right )=\lg {\left | {x} \right |}\)

D.\( f\left(x\right)={x}^{\frac{1}{3}}\)

参考答案：D

第414题

A.\( f\left(4\right)=-3\)

B.函数 \( f\left(x\right)\) 的图象与 \( x\) 轴有两个交点；

C.函数 \( y=f\left(x\right)\) 的最小值为 \( -4\)；

D.函数 \( y=f\left(x\right)\) 的最大值为 \( 4\)

参考答案：ABC

第415题

A.当 \( a=0,f\left(x\right)\) 的定义域为 \( (-\infty ,-1)\cup (1,+\infty )；\)

B.\( f\left(x\right)\) 一定有最小值；

C.当 \( a=0,f\left(x\right)\) 的值域为 \( R；\)

D.若 \( f\left(x\right)\) 在区间 \( [2,+\infty ）\)上单调递增，则实数 \( a\) 的取值范围是 \( \left\{a\right|a\ge -4\}\)

参考答案：AC

第416题

A.函数 \( f\left(x\right)\) 的图象关于 \( y\) 轴对称；

B.函数 \( f\left(x\right)\) 的最小值是 \( 2\)；

C.函数 \( f\left(x\right)\) 在 \( (0,+\infty )\) 上单调递增，在 \( (-\infty ,0)\) 上单调递减；

D.函数 \( f\left(x\right)\) 的单调递增区间是 \( (-\mathrm{1,0})\) 和 \( (1,+\infty )\)

参考答案：ABD

第417题

参考答案：\( \left(\mathrm{1,2}\right)\)

第418题

参考答案：\( [\frac{1}{2},8)\cup (8,+\infty )\)

第419题

参考答案：\( (-2,-1)\cup (-1,-\frac{1}{2})\cup (2,+\infty )\)

第420题

参考答案：\( (-\mathrm{1,2})\)