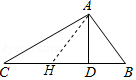
\(CD = BD + AB\)
证明:在\(CD\)上截取\(DH = DB\),连接\(AH\),
\(\because AD \bot BC\),
\(\therefore AB = AH\),
\(\therefore \angle AHB = \angle B\),
\(\because \angle B = 2\angle C\),
\(\therefore \angle AHB = \angle C\),
\(\because \angle AHB = \angle C + \angle HAC\),
\(\therefore \angle HAC = 2\angle C\),
\(\therefore AH = CH\),
\(\therefore AB = CH\),
\(\therefore AB + BD = CH + DH = CD\).