初中数学八年级下册（648题）

---

如图，在\({\rm{Rt}}\Delta ABC\)中，\(\angle BAC = 90^\circ \)，\(AD\)是边\(BC\)上的中线，过点\(A\)作\(AE//BC\)，过点\(D\)作\(DE//AB\)，\(DE\)与\(AC\)、\(AE\)分别交于点\(O\)、\(E\)，连接\(EC\)，求证：四边形\(ADCE\)是菱形。

    

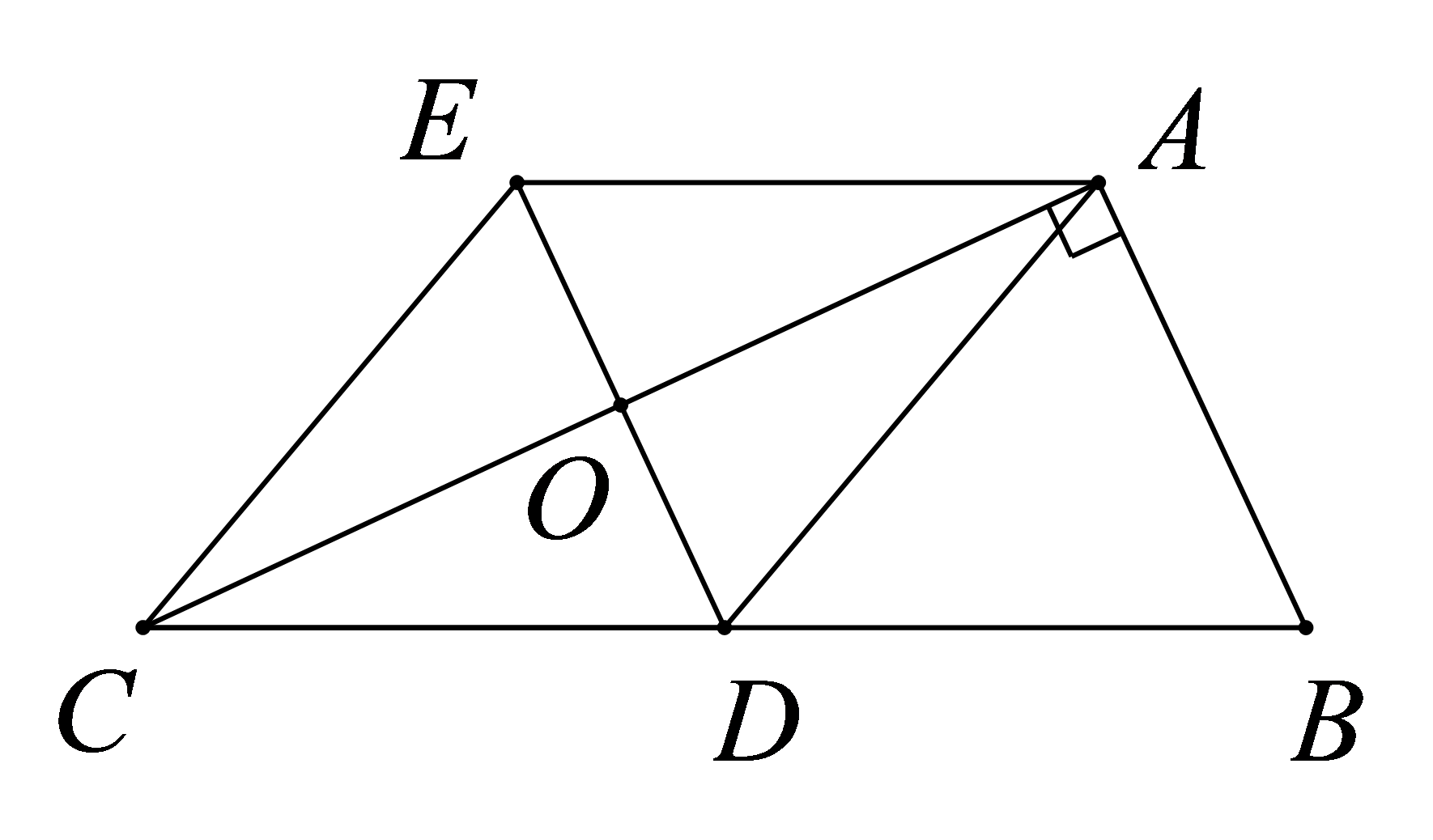
知识点：第十八章　平行四边形

参考答案：证明： \(\because AE//BC\)，\(DE//AB\)，

\(\therefore \)四边形\(ABDE\)为平行四边形；

\(\therefore \)\(AE = BD\)，

\(\because AD\)是边\(BC\)上的中线，

\(\therefore BD = CD\)，

\(\therefore AE = CD\)，

\(\because AE//CD\)

\(\therefore \)四边形\(ADCE\)是平行四边形，

又\(\because \angle BAC = 90^\circ \)，\(AD\)是边\(BC\)上的中线，

\(\therefore AD = \frac{1}{2}BC = CD\)，

\(\therefore \)平行四边形\(ADCE\)是菱形。