初中数学八年级下册（648题）

---

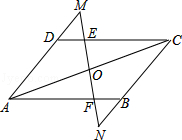
如图，\( O\)为\(▱ABCD\)对角线\( AC\)的中点，过\( O\)点作一直线与\( DC\mathrm{、}AB\)交于\( E\mathrm{、}F\)，并与\( AD\mathrm{、}CB\)的延长线分别交于\( M\mathrm{、}N\)．求证：\( DM＝BN\)。

知识点：第十八章　平行四边形

参考答案：证明：
\(\because ▱ABCD\mathrm{}，\)
\(\therefore AD=BC，\mathrm{}AD//BC，\)
\(\therefore \mathrm{\angle }DAC=\mathrm{\angle }ACB\)。

在\( △OAM\)和\( △OCN\)中，
\( \because \mathrm{\angle }DAC=\mathrm{\angle }ACB，OA=OC，\mathrm{\angle }AOE=\mathrm{\angle }COF，\)
\(\therefore △OAM≌△OCN（\mathrm{A}\mathrm{S}\mathrm{A}），\)
\(\therefore AM=CN\)

\( \because AD=BC，\therefore DM=BN\)。